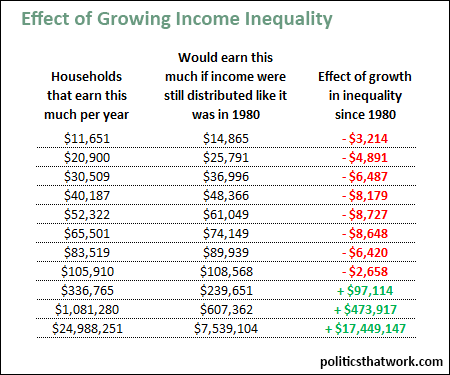
Description: This table shows the current household incomes of various percentiles for income in the left column, and what the income of people at that percentile would be if the distribution of income today were the same as it was in 1980 in the middle column. The right column shows the difference between what their income would be if the income distribution remained the same and what their income is today (i.e., a negative, red, number means that they are losing that much income to the increase in inequality). The rows represent the income of households at the 10th, 20th, 30th, 40th, 50th, 60th, 70th, 80th, 90th, 99th and 99.9th percentiles and the top 0.01% for income. Note that the 10th, 30th, 50th and 70th percentile figures are actually average figures for the quintiles, as the boundary figures are not available, and that the top 0.01% is the average for the top 0.01%.
The calculations are simple. I took the incomes at various percentiles as they stood in 1980, and applied the overall average increase in income of 28% to determine what those percentiles would earn today if the distribution had remained constant.
Sources: Census Census Saez
Data: Excel
Last updated: March 16, 2016





